I am writing this post for those who are going to appear for the GATE-2019. If you see the Previous Year Question Papers, you will find that finding RMS for a composite wave or for an arbitrary function is asked frequently. Therefore it is quite important to know “How to find RMS value”.
I will recommend you to always remember the basics and apply the basics while solving any problem. So here also we will start from basic without going into the definition of RMS value.
The RMS value of any function, say y= f(x) is given as,
The above formula is the very basic definition of RMS value and in case you do not find any way while solving problem related to RMS value then you must use the basic. I am going to show here, how to use this very basic definition of RMS value for solving problem.
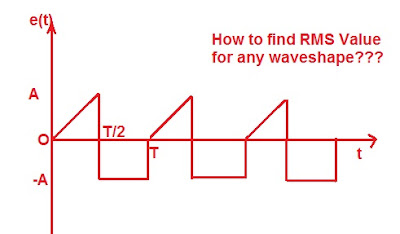
Suppose, we are asked to find the RMS value for the wave e(t), shown below.
So, we will use our basic to solve this problem. As can be seen from the waveform, the wave is in two different parts for a complete time period i.e. it has one equation in 0-T/2 and another equation from T/2-T. Therefore our integration will be broken into two integrals i.e. one from 0-T/2 and another from T/2-T.
But first of all we need to get the equation of the waveform for the two intervals.
For time t = 0 – T/2,
See it is a straight line passing through origin, so let y =mx
But at t = T/2, y = A
So, A = mxT/2
i.e. m = 2A/T
Hence, y = 2Ax/T for t = 0 – T/2
For time t = T/2 – T,
y = -A
Thus we know the equation of lines for the intervals. Now, we will proceed to calculate the RMS value.
You will think that it is lengthier but honestly speaking it will really help you and once you have practice, this won’t take much of your time. And the most important thing is that you need not to remember too many things like area under the curve etc…just remember the basic…that’s all…
Next suppose you are given a complex wave having harmonics like,
I = ID +I1mSin(ωt-Ø) + I2mSin(2ωt-Ø) + I3mSin(3ωt-Ø) +……… InmSin(nωt-Ø)
In such case,
RMS value
= ID + Square Root of individual RMS values
It is strongly recommended to solve IES Question Bank objectives only. Do not solve IES Question Bank subjective.
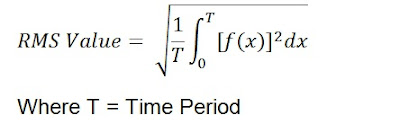
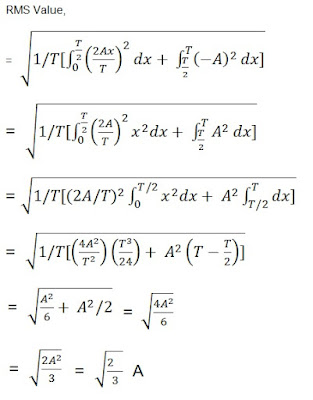
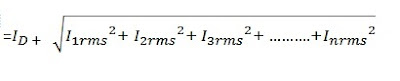
Please provide example for second type questions….🙏🙏
Sure…please check latter..I will add example