Statement of Substitution Theorem:
Substitution Theorem states that any branch of a DC bilateral circuit can be substituted by a combination of various circuit elements, provided the current & voltage across the branch remains unchanged. Basically, this theorem tells us the boundary condition to replace a circuit branch.
If the value of current and voltage across a branch of circuit network is known, then we can replace that branch by other circuit element like current source, voltage source etc. in such a way that the current & voltage across that branch remain the same. This replacement is called the branch equivalent.
In simple words, the statement of Substitution Theorem may be defined as: the necessary condition for branch equivalence of a network is that current & voltage across it must not change. The limitation of this theorem is that it cannot be used to solve network containing two or more sources which are not in series or parallel.
Explanation:
Let us consider a circuit as shown below. Let us assume that we want to apply substitution theorem for branch x-y. This means, we want to replace this branch by its equivalent branch.
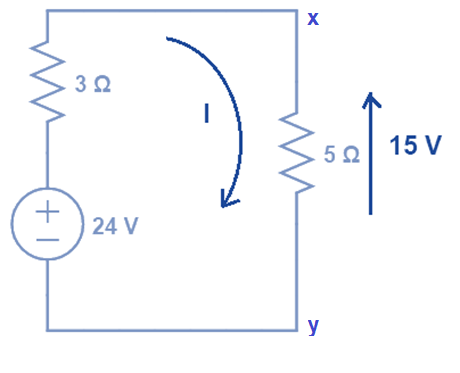
Before we substitute branch x-y, we need to know the current & voltage across it. Let us find this.
Current I through branch x-y
= 24/8 = 3 A
Voltage across branch x-y
= 5×3
= 15 V
Thus, the current and voltage across the branch is 3 A & 15 V respectively. Knowledge of these two parameters is the very first requirement of Substitution Theorem.
Now, we have to replace the branch x-y by some other circuit element while keeping the current & voltage to be 3 A and 15 V respectively. This can be done in a number of way which is shown in figure below.

Carefully observe (1), it is a series combination of 2.5 Ω resistance and a cell of voltage 7.5 V. This is an equivalent branch of x-y. This is because, when (1) is connected across terminals x-y, the current through it will be 3 A and hence the voltage drop will be 15 V. Thus, the value of current and voltage across x-y remains unchanged. Therefore, according to Substitution Theorem, circuit element (1) represents equivalent branch of x-y.
You may ask why voltage and resistance have been chosen 2.5 V and 7.5 V in element (1). These values are so chosen that the current & voltage across the terminals of element (1) remains 3 A and 15 Volt. In fact, there may infinite number of values of resistance and voltage possible provided the binding condition remain the same. Here binding condition is to keep the terminal voltage 15 V and current 3 A. Thus, branch x-y may be substituted by an infinite number of equivalent branches.
Similarly, you may check that element (2), (3) and (4) are the equivalent branch. Aren’t they? Kindly write in comment box.